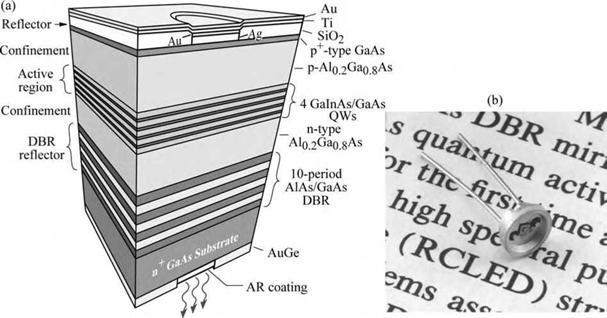
The structure of an RCLED with a GalnAs active region is shown in Fig. 15.4 (a). The cavity is defined by one distributed Bragg reflector (DBR) and one metallic reflector. Also included are two confinement regions and a four-quantum-well active region. The heavily doped n-type substrate is coated with a ZrO2 anti-reflection layer (Schubert et al., 1994). A picture of the first RCLED is shown in Fig. 15.4 (b).

The motivation for the metal reflector is twofold. Firstly, the metallic Ag reflector serves as a non-alloyed ohmic contact to the heavily doped p-type (NA « 5 x 1019 cm-3) GaAs top layer, thus effectively confining the pumped region to the area below the contact. Secondly, it was shown in the preceding section that the cavity length must be kept as short as possible for maximizing the emission enhancement. Owing to the lack of a penetration depth, metal reflectors allow for a short cavity length. Cavities with two metallic reflectors have been reported (Wilkinson et al.,

1995) . However, optical absorption losses in the light-exit mirror can be large in a double metal mirror structure, unless very thin metallic reflectors are used (Tu et al., 1990). The lack of a p - type DBR also avoids the well-known problem of high resistance in p-type DBRs (Schubert et al., 1992c; Lear and Schneider, 1996). It has been shown that parabolic grading yields the lowest ohmic resistance in DBRs. Such parabolic grading is suited to eliminating heterojunction band discontinuities (Schubert et al., 1992c).

The magnitude of the reflectivity of the DBR needs to be consistent with Eqs. (15.1) and (15.3). The Ag back mirror has a reflectivity of approximately 96%. According to Eq. (15.1), the DBR reflectivity must be < 96%. The second criterion of Eq. (15.3) requires that 2 £ a Lactive < 1 - R1. Assuming £ = 1.3, a = 104 cm-1, Lactive = 400 A, one obtains the condition R1 < 90%. Thus the mirror reflectivity of RCLEDs must be much lower than that of VCSELs. A high reflectivity would increase self-absorption and decrease the light output of the device as discussed earlier. De Neve et al. (1995) used an extensive theoretical model to calculate the mirror reflectivity. The maximum efficiency was calculated at a reflectivity of R1 = 50-60%.

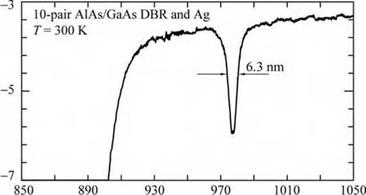
The reflection and emission properties of the RCLED are shown in Figs. 15.5 (a) and (b). The reflection spectrum of the RCLED (Fig. 15.5 (a)) exhibits a highly reflective band for wavelengths > 900 nm and a dip in the reflectivity at the cavity resonance. The spectral width of the cavity resonance is 6.3 nm. The emission spectrum of an electrically pumped device, shown in Fig. 15.5 (b), has nearly the same shape and width as the cavity resonance.

In conventional LEDs, the spectral characteristics of the devices reflect the thermal distribution of electrons and holes in the conduction and valence bands. The spectral characteristics of light emission from microcavities are as intriguing as they are complex. However, restricting our considerations to the optical axis of the cavity simplifies the cavity physics considerably. If we assume that the cavity resonance is much narrower than the natural emission spectrum of the semiconductor, then the on-resonance luminescence is enhanced whereas the off-resonance luminescence is suppressed. The on-axis emission spectrum should therefore reflect the enhancement, that is, the resonance spectrum of the cavity. The experimental results shown in Fig. 15.5 confirm this conjecture.

(a)

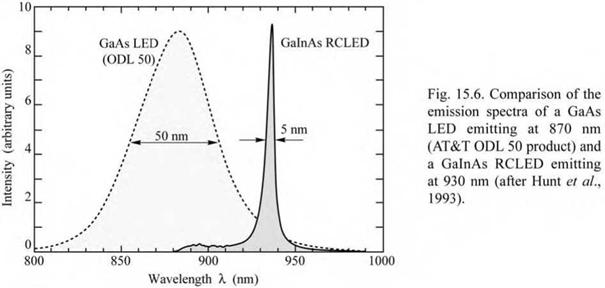
Owing to the cavity, the emission spectrum of an RCLED is much narrower than the emission spectrum of regular LEDs (Schubert et al. 1992a; Hunt et al., 1992, 1993). The spectral width of the RCLED emitted into a certain direction is given by the optical characteristics of the cavity. In contrast, the spectral width of a regular LED is about 1.8 kT, a value that is much wider than the RCLED emission spectrum. A comparison of a regular GaAs LED and a GaInAs

RCLED emission spectrum is shown in Fig. 15.6. Comparison of the spectra shows that the RCLED emission spectrum is a factor of about 10 narrower than the spectrum of the GaAs LED.

A regular LED has little or no angle dependence of the emission spectrum. However, the reflective properties of DBRs and of cavities consisting of DBRs are angle-dependent. Consequently, the emission from an RCLED along a certain direction is narrower than that of a regular LED. Integrated over all directions, the RCLED has a broad emission spectrum.

A figure of merit for LEDs used in optical fiber communication systems is the photon flux density emitted from the diode at a given current, which, for a given wavelength, can be characterized in terms of the unit microwatts per steradian. The optical power coupled into a fiber is directly proportional to the photon flux density.

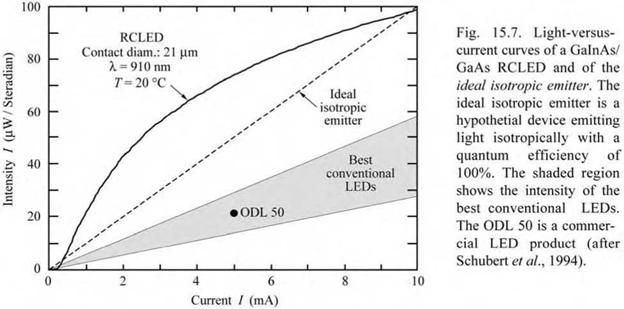
The intensity of an RCLED as a function of the injection current is shown in Fig. 15.7. For comparison, the calculated intensity of the ideal isotropic emitter, which is a hypothetical device, is also shown. The ideal isotropic emitter is assumed to have an internal quantum efficiency of 100% and the device is assumed to be clad by an anti-reflection coating providing zero reflectivity (R = 0) for all wavelengths emitted from the active region. If the photon emission inside the semiconductor is isotropic, then the optical power per unit current per unit solid angle normal to the planar semiconductor surface is given by

^optical _ 1 hc (15 4)

_ 2 л (154)

4n n e X

where Q represents the unit solid angle, n is the refractive index of the semiconductor, c is the velocity of light, e is the electronic charge, and X is the emission wavelength in vacuum. Equation (15.4) is represented by the dashed line in Fig. 15.7. Neither the 100% internal quantum efficiency nor the hypothetical anti-reflection coating can be reproduced in practice for fundamental reasons. Therefore, the ideal isotropic emitter represents an upper limit for the intensity attainable with any conventional LED. Of course, even the best conventional LEDs have intensities lower than that of the ideal isotropic emitter. Also included in Fig. 15.7 is the ODL 50 GaAs LED, frequently used for optical fiber communication. All devices shown in Fig. 15.7 have planar light-emitting surfaces, and no lensing is used.

Figure 15.7 reveals that the RCLED provides unprecedented intensities in terms of both absolute values and slope efficiencies. The slope efficiency is 7.3 times the efficiency of the best conventional LEDs and 3.1 times the calculated efficiency of the ideal isotropic emitter. At a current of 5 mA, the intensity of the RCLED is 3.3 times that of the best conventional LEDs including the ODL 50. The high efficiencies make the RCLED well suited for optical interconnect and communication systems.

The higher spectral purity of RCLEDs reduces chromatic dispersion in optical fiber communications (Hunt et al., 1993). The chromatic dispersion is directly proportional to the linewidth of the source. Since RCLEDs have linewidths 5-10 times narrower than conventional LEDs, chromatic dispersion effects, which dominate at wavelengths of 800-900 nm, are reduced as well. Hunt et al. (1993) showed that the bandwidth of RCLEDs is a factor of 5-10 higher than
that of conventional LEDs. An RCLED-versus-LED comparison in a transmission experiment is shown in Fig. 15.8. The results show the received signal after transmission lengths of 5 m and

3.4 km for the two devices. The fiber used is a graded-index multimode fiber with a core diameter of 62.5 ^m. After a transmission length of 5 m, no marked difference is found for the two devices. However, a substantial difference is found after a transmission length of 3.4 km. Inspection of Fig. 15.8 reveals that the RCLED exhibits much less pulse broadening as compared to the conventional LED. This difference is due to reduced material dispersion for the RCLED.

Schubert et al. (1996) demonstrated the high-speed modulation capability of RCLEDs. Eye diagram measurements with a random bit pattern generator revealed wide-open eyes at 622 Mbit/s. Due to the small size of the current injected region, the parasitic capacitances of communication RCLEDs are small. It is expected that RCLEDs will be suitable for modulation frequencies exceeding 1 Gbit/s.

Fig. 15.8. Signal detected at the receiver end of a graded - index multimode fiber with a core diameter of 62.5 ціп using (a) a GalnAs RCLED and (b) a GaAs LED source. After a short transmission dis­tance of 5 m, no marked dif­ference is found for the two sources. After a trans-mission distance of 3.4 km, the RCLED exhibits much less pulse broadening than the LED (after Hunt et al., 1993).